views
Predicting a Number (Easy)
Ask your friend to pick a number. They can pick any number, but tell them to keep it a secret from you! For this example, we’ll say your friend picked the number 6. To make the trick easier for you, you might tell them to pick a number between 1-9.

Tell your friend to double the number. Say, "Now double the number you chose, but don't tell me the answer." You might also say, “Multiply your number by 2,” which is the same as doubling it. In our example, 6 x 2 = 12.
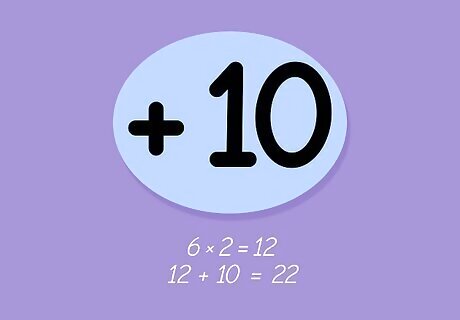
Tell your friend to add 10 to their doubled number. Ask them to add 10 to their last answer. Remind them to keep it a secret! 12 + 10 = 22

Ask them to divide the answer by 2. Tell your friend you're trying to read their mind, but the number is too big right now. Ask them to divide the answer by 2 to make it smaller. 22 ÷ 2 = 11.

Tell them to subtract their original number from their current number. Say, “Now take away your original number from your last answer.” The person in our example picked 6, so they would calculate 11 - 6 = 5.

Announce that the answer is 5! Point to your forehead and pretend to think very hard. Then say, “The number you have now is… 5!” If there are other people in the room, have your friend show them the piece of paper to prove it. This works because after you double the first number, divide it by 2, and subtract the original number, you've canceled out the original number completely. But the extra numbers and instructions you gave him always come out to 5!
The 37 Trick (Intermediate)

Tell your friend to write down the same digit 3 times. Ask them to choose any number between 1 and 9, and to write it 3 times in a row. Remind them to keep it a secret! Also, grab a calculator—it’ll come in handy. For our example, we’ve chosen 555.

Instruct them to add all three digits together. Tell your friend to take the three digits they wrote down and add them together to find a new number. In this example, 5 + 5 + 5 = 15.
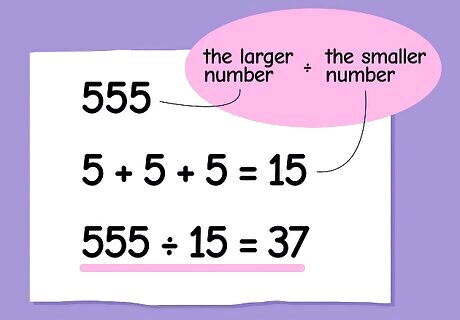
Tell them to divide the larger number by the smaller one. Your friend now has two numbers written down: a three-digit number and a smaller one. Ask them to take the three-digit number and divide it by the smaller one they just found by adding. 555 ÷ 15 = 37.

Announce that they're thinking of the number 37. Whether they chose 111, or 999, or one of the options in between, your volunteer will always end up with 37 as their answer. You just read their mind with math!
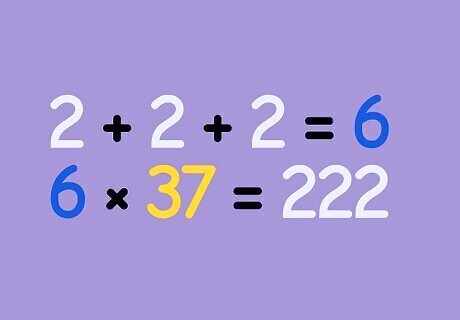
Reveal that the trick uses basic arithmetic. There's no magic here, just simple math! When you add three identical single-digit numbers together, then multiply the answer by 37, you get a number that’s just those three digits in a row. In this trick, you’re using division to prove this, only backward! Try it with any other single-digit number. For example: 2. 2 + 2 + 2 = 6, and 6 x 37 = 222. If your friend used “2” as their starting number, they’d divide 222 by 6 and get 37!
The Gray Elephant from Denmark Trick (Intermediate)

Write the phrase, “Gray elephant from Denmark” on paper. Tell your friend that you're writing their future thoughts down on your slip of paper, but keep what’s on the paper secret! We’ll use some trickery and math to make sure they think “gray elephant from Denmark.”

Tell your friend to multiply a number from 2-10 by 9. They can select any single number between 2 and 10. Then, they multiply it by the number 9. For our example, the friend picked 4. 4 x 9 = 36.

Ask your friend to add the digits of the new number together. Any number multiplied by 9 is a multiple of 9. Also, when they add the 2 digits of their number together, it will always be 9. 3 + 6 = 9
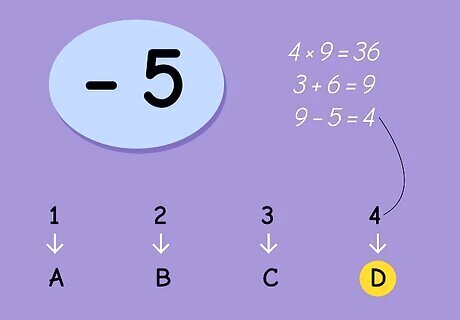
Tell them to subtract 5 from their answer, then assign them a letter. Tell them that if their answer is 1, their letter is A. If their answer is 2, their letter is B. If their answer is 3, their letter is C, and if their answer is 4, their letter is D. This is all just to distract them. Since their number was always going to be 9, and 9 - 5 = 4, their letter will always be D.
Ask them to think of a country in Europe that begins with their letter. Since Denmark is the only country in Europe that starts with D, their answer will always be Denmark! Let them search the internet for an answer, if they don’t know off the top of their head.
Tell them to think of a mammal that starts with the 2nd letter of their country. Since mammals don’t include birds or fish, their answer will most likely be “elephant.”

Ask them to think about the color of their animal. They’ll most likely think, “gray.” Have you ever seen an elephant that wasn’t gray?

Reveal your slip of paper with the writing on it. Watch their eyes go wide as you reveal that you predicted their exact thoughts! This trick might not always work if your friends are especially creative, though. You might add a time limit to put some pressure on them and make them think of the most likely answers.
Guessing The Secret Digit (Advanced)

Hand a calculator, pencil, and paper to a volunteer. This trick involves large numbers, so a calculator that has plenty of space will come in handy. Tell your friend that you'll be reading their mind, so they must keep everything on the paper and calculator hidden from you. This trick also requires some quick thinking on your part. It's nothing harder than addition, but you'll need to be fast and error-free.

Tell them to write down three positive, consecutive numbers. These can be any whole numbers, but they must be consecutive, meaning they come one right after the other, there can be no whole numbers between them. For our example, a volunteer picked the numbers 19, 20, and 21. Not that if the calculator only has room to display eight digits, the numbers must all be between 1 and 21. If it only has room to display six digits, make it 1 to 9.
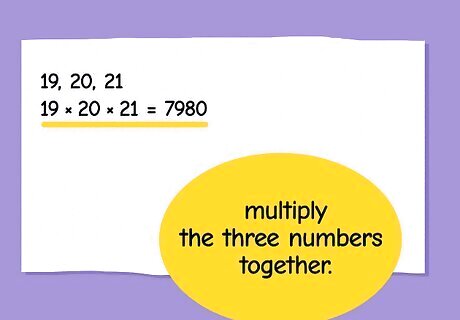
Ask them to multiply the three numbers together. After they find the answer on the calculator, tell them to write it down on the piece of paper. In our example, 19 x 20 x 21 = 7980.
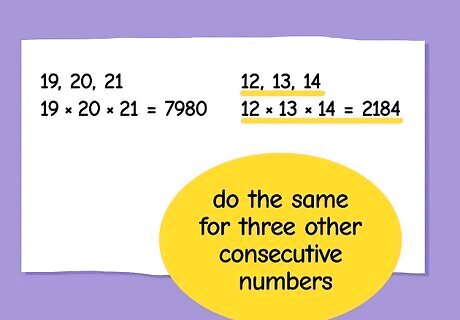
Tell the volunteer to do the same for three other consecutive numbers. Next, the person you're "mind reading" secretly picks three more consecutive numbers. They multiply them together, as before, and write them down next to his first answer. Let's say they pick 12, 13, 14. They then multiply 12 x 13 x 14 = 2184.
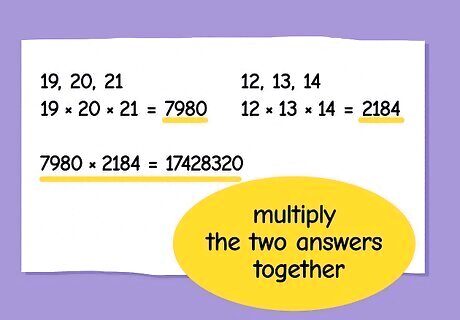
Ask them to multiply the two answers together. Now the volunteer multiplies his two products together and writes down the result on the piece of paper. 7980 x 2184 = 17428320.
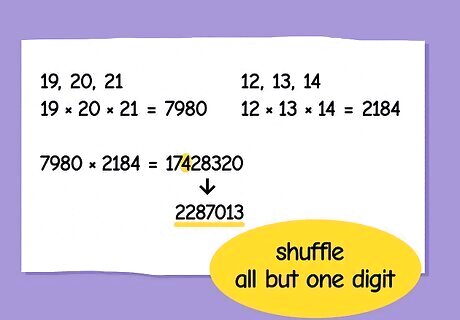
Tell the volunteer to shuffle all but one digit. Explain that you only have enough power to read one of the digits from their mind. Ask them to pick one secret digit in the number, then write down the other digits after shuffling them in any order. For example, if they pick 4 as the secret digit, they could shuffle the rest of the digits as 2287013.
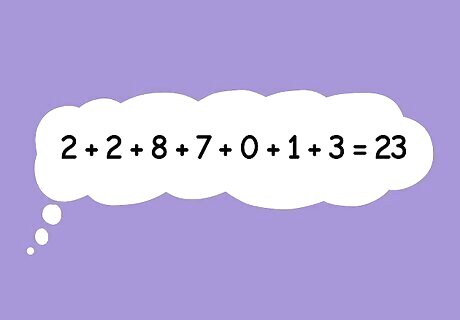
Add the shuffled digits in your head as the volunteer reads them aloud. Remind them that they must keep one digit secret. Have them read the other digits slowly and clearly while you add them in your head. For example, they would read "2... 2... 8... 7... 0... 1... 3" while you add these together as they go, getting a total of 23.
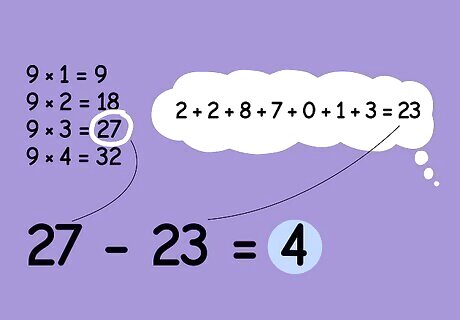
Find the secret digit in your head. All of the digits their answer, including the secret one, will add up to a multiple of 9. Once you know this, there are two ways to find the secret digit. Either find the next multiple of 9, then subtract the sum from that multiple; or, add the digits of the sum, find the next multiple of 9 from the sum, then subtract the new sum from that multiple. For our example: The non-secret digits add up to 23, and you know the next multiple of 9 is 27. Think 27 - 23 = 4, so the last digit must be 4. Or, if you're not sure what the next multiple of 9 is, add the digits in your sum and repeat. For example, 23 → 2 + 3 = 5. Now the next multiple of 9 is 9, and 9 - 5 = 4.
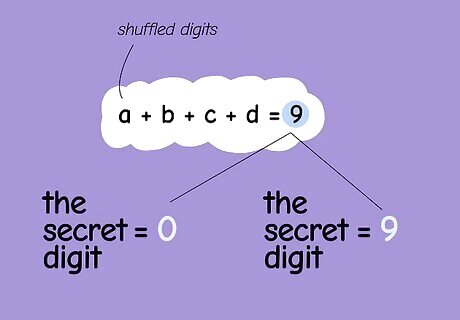
Guess either 0 or 9 if the shuffled digits add up to 9. If you add the digits they spoke out loud and you already have a multiple of 9, there are two possibilities. Either the secret digit is 0, or the secret digit is 9. Make a guess at one of them to read their mind. If you get it wrong, make a joke ("I knew I should have studied harder in telepathy school!"), then guess again with the second possibility.
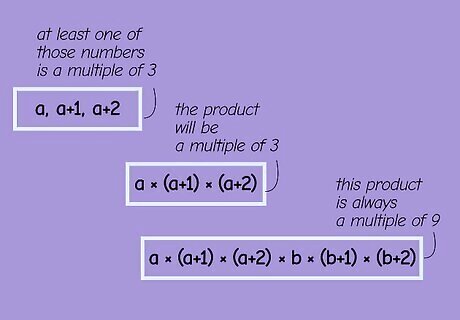
Reveal that the trick depends on multiples of 3. Any number that adds up to a multiple of 3 is also a multiple of 3. Also, any three consecutive numbers includes a multiple of 3, so the product of the trio will also be a multiple of 3. Since your volunteer multiplies three consecutive numbers together, the answer itself will be a multiple of three. Further, this product is always a multiple of 9 (since 3 x 3 = 9). All multiples of 9 have the property described above, where all of their digits sum to another multiple of 9.


















Comments
0 comment