How to Memorize the Quadratic Formula

Understanding the Formula

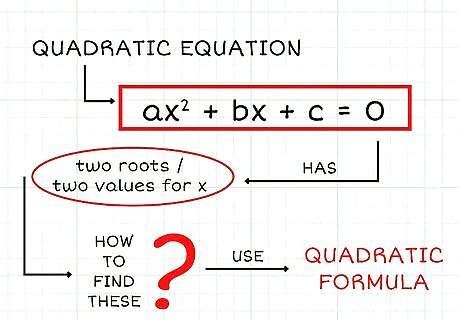
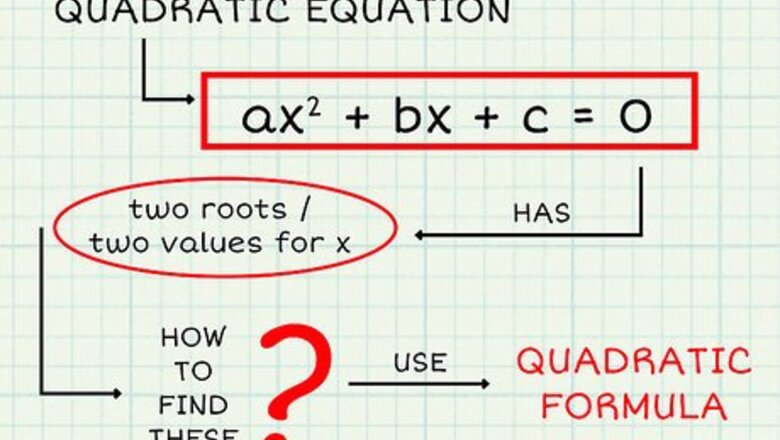
Know what the formula is used for. The formula is used to find the value of x {\displaystyle x} x in a quadratic equation. A quadratic equation takes the form of a x 2 + b x + c = 0 {\displaystyle ax^{2}+bx+c=0} ax^{{2}}+bx+c=0. Remember that a quadratic equation will have two roots (two values for x {\displaystyle x} x). Using the quadratic formula will provide you with these roots. Understanding the purpose of a complicated math formula makes it much easier to remember.

Write down the formula. The formula is x = − b ± b 2 − 4 a c 2 a {\displaystyle x={\frac {-b\pm {\sqrt {b^{2}-4ac}}}{2a}}} x={\frac {-b\pm {\sqrt {b^{{2}}-4ac}}}{2a}}, where the variables a {\displaystyle a} a, b {\displaystyle b} b, and c {\displaystyle c} c are derived from the coefficients in the quadratic equation a x 2 + b x + c = 0 {\displaystyle ax^{2}+bx+c=0} ax^{{2}}+bx+c=0. Plug the values of these variables into the formula. For example, in the equation 4 x 2 + 6 x + 2 = 0 {\displaystyle 4x^{2}+6x+2=0} 4x^{{2}}+6x+2=0: a = 4 {\displaystyle a=4} a=4 b = 6 {\displaystyle b=6} b=6 c = 2 {\displaystyle c=2} c=2 Plugging these values into the equation: x = − 6 ± 6 2 − 4 ( 4 ) ( 2 ) 2 ( 4 ) {\displaystyle x={\frac {-6\pm {\sqrt {6^{2}-4(4)(2)}}}{2(4)}}} x={\frac {-6\pm {\sqrt {6^{{2}}-4(4)(2)}}}{2(4)}}

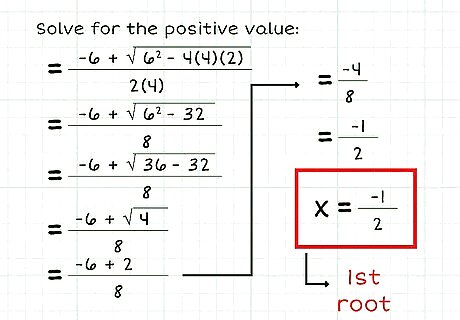
Solve for the positive value. This means solving the equation x = − b + b 2 − 4 a c 2 a {\displaystyle x={\frac {-b+{\sqrt {b^{2}-4ac}}}{2a}}} x={\frac {-b+{\sqrt {b^{{2}}-4ac}}}{2a}}. This will give you the first root of the equation. For example: x = − 6 + 6 2 − 4 ( 4 ) ( 2 ) 2 ( 4 ) {\displaystyle x={\frac {-6+{\sqrt {6^{2}-4(4)(2)}}}{2(4)}}} x={\frac {-6+{\sqrt {6^{{2}}-4(4)(2)}}}{2(4)}} x = − 6 + 6 2 − 32 8 {\displaystyle x={\frac {-6+{\sqrt {6^{2}-32}}}{8}}} x={\frac {-6+{\sqrt {6^{{2}}-32}}}{8}} x = − 6 + 36 − 32 8 {\displaystyle x={\frac {-6+{\sqrt {36-32}}}{8}}} x={\frac {-6+{\sqrt {36-32}}}{8}} x = − 6 + 4 8 {\displaystyle x={\frac {-6+{\sqrt {4}}}{8}}} x={\frac {-6+{\sqrt {4}}}{8}} x = − 6 + 2 8 {\displaystyle x={\frac {-6+2}{8}}} x={\frac {-6+2}{8}} x = − 4 8 {\displaystyle x={\frac {-4}{8}}} x={\frac {-4}{8}} x = − 1 2 {\displaystyle x={\frac {-1}{2}}} x={\frac {-1}{2}}So, the first root of the equation is x = − 1 2 {\displaystyle x={\frac {-1}{2}}} x={\frac {-1}{2}}

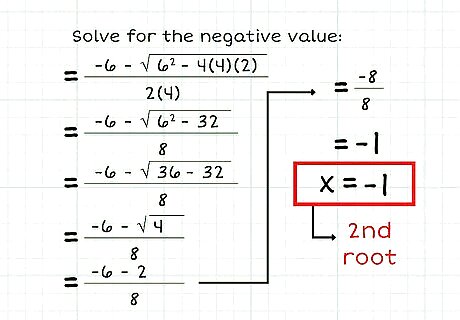
Solve for the negative value. This means solving the equation x = − b − b 2 − 4 a c 2 a {\displaystyle x={\frac {-b-{\sqrt {b^{2}-4ac}}}{2a}}} x={\frac {-b-{\sqrt {b^{{2}}-4ac}}}{2a}}. This will give you the first root of the equation. For example: x = − 6 − 6 2 − 4 ( 4 ) ( 2 ) 2 ( 4 ) {\displaystyle x={\frac {-6-{\sqrt {6^{2}-4(4)(2)}}}{2(4)}}} x={\frac {-6-{\sqrt {6^{{2}}-4(4)(2)}}}{2(4)}} x = − 6 − 6 2 − 32 8 {\displaystyle x={\frac {-6-{\sqrt {6^{2}-32}}}{8}}} x={\frac {-6-{\sqrt {6^{{2}}-32}}}{8}} x = − 6 − 36 − 32 8 {\displaystyle x={\frac {-6-{\sqrt {36-32}}}{8}}} x={\frac {-6-{\sqrt {36-32}}}{8}} x = − 6 − 4 8 {\displaystyle x={\frac {-6-{\sqrt {4}}}{8}}} x={\frac {-6-{\sqrt {4}}}{8}} x = − 6 − 2 8 {\displaystyle x={\frac {-6-2}{8}}} x={\frac {-6-2}{8}} x = − 8 8 {\displaystyle x={\frac {-8}{8}}} x={\frac {-8}{8}} x = − 1 {\displaystyle x=-1} x=-1So, the first root of the equation is x = − 1 {\displaystyle x=-1} x=-1

Using Music Mnemonics

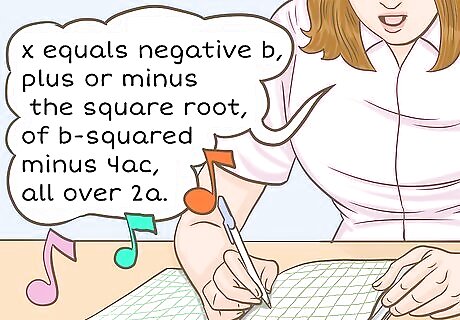
Sing to the tune of “Pop Goes the Weasel.” If you don't remember this song, it is the one that begins “Ring around the mulberry bush….” This is a common song and lyric used to remember the quadratic formula, and it is quick and easy to memorize. The lyrics are:x equals negative b,plus or minus the square root,of b-squared minus 4ac,all over 2a.

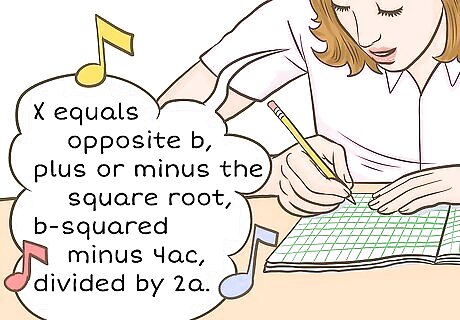
Sing to the tune of “Row, Row, Row Your Boat.” Most people know this song and already have the tune memorized. It is a catchy song that can easily get stuck in your head, which makes it ideal for helping to memorize the formula. The lyrics are:X equals opposite b,plus or minus the square root,b-squared minus 4ac,divided by 2a.

Make up your own song. This method can be especially effective if you pick a tune that is meaningful to you and create your own lyrics for it. You can make the song as silly or catchy as you want, but make sure to not make it too complicated, or it will be difficult to remember. Write down the lyrics of the song so that you remember it. Even better, make a video of yourself singing it. Then you can listen to the video during your study sessions and sing along with it. You could also post it to YouTube or another website so that it can help others who are also trying to remember the formula.

Using Word and Image Mnemonics

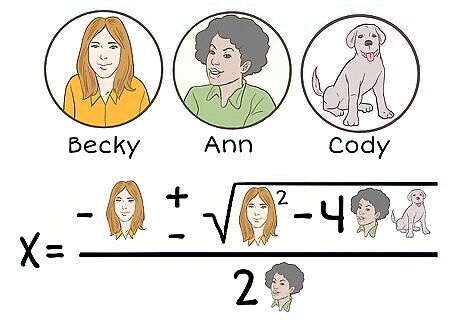
Create an expression mnemonic associating familiar names and numbers. Use names of famous people, or people you know, that begin with the letter of the variable. For example, if your sister's name is Becky, your friend's name is Ann, and your dog's name is Cody, you can memorize “Negative Becky, plus or minus the square root of Becky squared minus 4 Ann-Cody, over 2 Anns.”

Invent a story. Associate all of the symbols, numbers, and variables with images and actions that help you remember the formula. Inventing a unique, silly story can help memorize quickly. This method is good for visual learners, as it will help you make a visual representation of the formula. For example, you can associate a negative/minus sign with being sad, and a positive/plus sign with being happy. You can associate a radical sign with an umbrella, and a fraction with a subway. The variables can stand for the first letter in the name of a person or animal. For example, for the quadratic formula you could make up the following story: “A sad bison approached an umbrella that could have been happy or sad. Underneath the umbrella was a square bison and four sad alley cats. They all stood over a subway where 2 aardvarks were dancing.” Write down your story so that you can re-read it as you try to memorize the formula.

Create name mnemonics. Come up with silly sounding names or phrases that can help you remember each part of the formula. It is extra helpful to visualize what that person or thing might look like. Coming up with your own names and images can help the mnemonic stick by making it meaningful for you. For example, to remember the first part of the formula, − b ± {\displaystyle -b\pm } -b\pm , you could use the name “Negbee, Prime Minister” and visualize what such a leader might look like. To remember b 2 {\displaystyle {\sqrt {b^{2}}}} {\sqrt {b^{{2}}}}, you could say the phrase “Square Root Beer Square” and visualize a square made out of Root Beer wearing glasses.